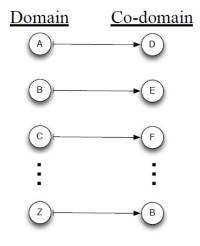
Definition

For each input element in a set <math>A</math> , a function assigns a single output element from another set <math>B</math> .

  • <math>A</math> is called the domain of the function
  • <math>B</math> is called the co-domain

!!!!!! Same as Set Theory - Function (Mathematical) !!!!!!

Syntax

<math>f(A) = B</math>

or in Mathese:

<math>f : A \rightarrow B</math>

Example

  • The function that doubles its input:
f({1, 2, 3,...}) = {(1,2),(2,4),(3,6),(4,8),....}
  • The function that multiplies the numbers forming its input:
f({1, 2, 3,...}x{1, 2, 3,...}) = {((1,1),2),((1,2),4),...,((2,2),4),(2,3),6), ....}
  • Caesar’s Cryptosystem

Function Domain Codomain Caesar Crypto

Each letter is mapped to one three places ahead, wrapping around, so MATRIX would map to PDWULA. The function mapping letter to letter can be written as: 

{('A','D'),('B','E'),('C','F'),('D','G'),...,('W','Z'),('X','A'),('Y','B'),('Z','C')}

Both the domain and the co-domain are {A,B, …,Z}

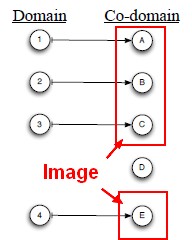
Image

for a function <math>f : V \mapsto W</math> , the image of f is the set of all images of elements of the domain: <MATH> {f (v) : v \in V} </MATH>

The image of a function is the set of all images of inputs. Mathese: 

lm f

The output of a given input is called the image of that input. The image of A under the function f is denoted f(A).

There might be elements of the co-domain that are not images of any elements of the domain.

Example
A function f : {1, 2, 3, 4} −> {'A','B','C','D','E'}
The image of f is lm f = {'A','B','C','E'}
'D' is in the co-domain but not in the image		 Domain Codomain Image

Set

Set of functions: For sets F and D, <math>F^D</math> denotes all functions from D to F.

For finite sets, the cardinality <math>|F^D| = |F|^{|D|}</math>

Function

Identity

Function - Identity

Composition

Linear Algebra - Function composition

Inverse

Functions f and g are functional inverses if f circ g and g circ f are defined and are identity functions.

Invertible

A function that has an inverse is invertible.

Invertible functions are:

Function Invertibility Theorem: A function f is invertible if and only if it is one-to-one and onto.

Linear-Function Invertibility Theorem: A function <math>f: V \mapsto W</math> is invertible iff

  • <math>\text{dim Ker f = 0 and dim lm f = dim W }</math>
  • <math>\text{dim Ker f = 0 and dim V = dim W }</math> (For f to be invertible, need dim V = dim W)

Extracting an invertible function:

Let <math>A = \begin{bmatrix} \begin{array}{r|r|r} 1 & 2 & 1 \\ 2 & 1 & 1 \\ 1 & 2 & 1 \\ \end{array} \end{bmatrix} </math> and define <math>f : \mathbb{R}^3 \mapsto \mathbb{R}^3</math> by <math>f (x) = Ax</math>

Define <math>W^* = lm f = Col A = Span \{[1, 2, 1], [2, 1, 2], [1, 1, 1]\}</math>

One basis for <math>W^*</math> is:

  • <math>w_1 = [0, 1, 0]</math> ,
  • <math>w_2 = [1, 0, 1]</math>

Pre-images for <math>w_1</math> and <math>w_2</math> :

  • <math>v_1 = [\frac{1}{2},−\frac{1}{2},\frac{1}{2}]</math>
  • and <math>v_2 = [−\frac{1}{2},\frac{1}{2},\frac{1}{2}]</math>

for then <math>A.v_1 = w_1</math> and <math>A.v_2 = w_2</math>

Let <math>V^* = Span \{v_1, v_2\}</math> Then <math>f : V^* \mapsto lm f </math> is onto and one-to-one and therefore invertible.

Linear

Linear Algebra - Linear Function (Weighted sum)

Relationship

One-to-one

Function Not One To One

<math>f : V \rightarrow W</math> is one-to-one if f (x) = f (y) implies x = y

One-to-One Lemma: A linear function is one-to-one if and only if its kernel is a trivial vector space. Equivalent: if its kernel has dimension zero.

f is one-to-one iff <math>\href{dimension}{dim} \text{ } \href{#kernel}{Ker} \text{ } f = 0</math>

Onto

Function Not Onto

<math>f : V \rightarrow W</math> is onto if for every <math>z \in F</math> there exists an a such that <math>f(a) = z</math>

f is onto if its image equals its co-domain

For any linear function <math>f : V \mapsto W</math> , f is onto if <math>\href{dimension}{dim} \text{ } \href{#image}{lm} f = \href{dimension}{dim} \text{ } W</math>